2023-02-14 スイス連邦工科大学ローザンヌ校(EPFL)
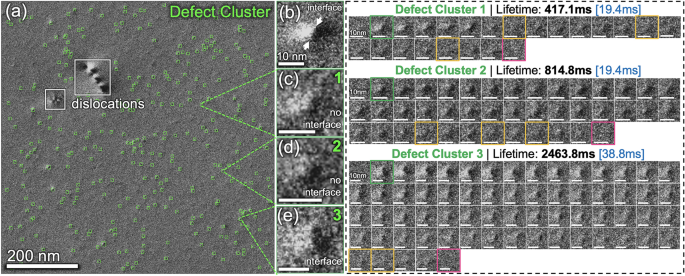
◆EPFLのエルゴード幾何学群理論研究ユニットを率いるニコラス・モノー教授は、「DNAのような複雑なものが究極的には単純な原子からできているように、重要な情報を含んでいるのは、その単純な断片そのものよりも、その組み立て方なのです」と語っています。モノー教授は、パデュー大学の研究者らと共同で、球体に関する古くからの疑問を解決した。その答えは、有力な数学雑誌『Inventiones』に掲載された。
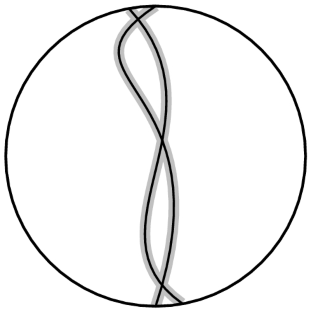
◆1958年、フィールズ賞メダリストのジョン・ミルナーは、円と2次元曲面だけを使って空間を作ろうとしたときに、ある問題に気がついた。この発見は、高次元の研究分野全体に雪だるま式に広がり、数学者たちは、ミルナーの「複雑さの限界」がすべての次元の空間に適用されるわけではないことにすぐに気がついたのである。
◆空間を理解する古典的なアプローチでは、この4次元の問題を解決できないことが証明されています。そこでEPFLの数学者たちは、スイスの数学者ヤコブ・ベルヌーイにちなんで名づけられたベルヌーイ過程にヒントを得たのである。ベルヌーイ過程は、コインを投げるモデルであり、球体やオイラー類の研究と組み合わせることで、最終的にこの問題を解決することができた。
<関連情報>
- https://actu.epfl.ch/news/tossing-coins-to-understand-spheres/
- https://link.springer.com/article/10.1007/s00222-023-01181-w
同相群および微分同相群の有界・無界コホモロジー Bounded and unbounded cohomology of homeomorphism and diffeomorphism groups
Nicolas Monod & Sam Nariman
Inventiones Mathematicae Published:06 February 2023
DOI:https://doi.org/10.1007/s00222-023-01181-w
Abstract
We determine the bounded cohomology of the group of homeomorphisms of certain low-dimensional manifolds. In particular, for the group of orientation-preserving homeomorphisms of the circle and of the closed 2-disc, it is isomorphic to the polynomial ring generated by the bounded Euler class. These seem to be the first examples of groups for which the entire bounded cohomology can be described without being trivial. We further prove that the Cr-diffeomorphisms groups of the circle and of the closed 2-disc have the same bounded cohomology as their homeomorphism groups, so that both differ from the ordinary cohomology of Cr-diffeomorphisms when r>1>1. Finally, we determine the low-dimensional bounded cohomology of homeo- and diffeomorphism of the spheres Sn and of certain 3-manifolds. In particular, we answer a question of Ghys by showing that the Euler class in H4(Homeo∘(S3))4(Homeo∘(3)) is unbounded.