2025-04-07 ハーバード大学
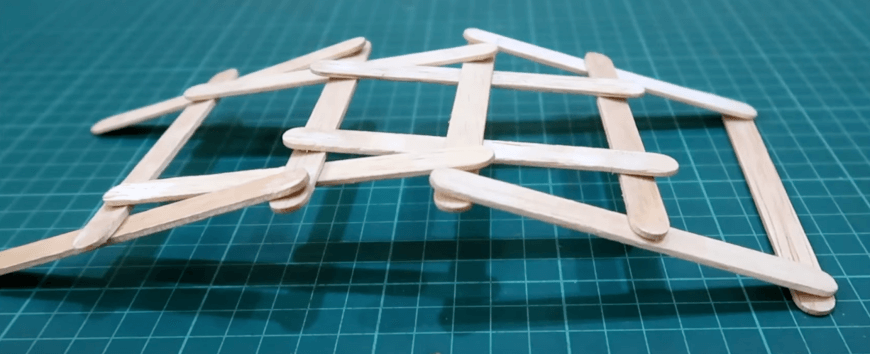
An example of da Vinci’s self-supporting bridge made out of popsicle sticks.
<関連情報>
- https://seas.harvard.edu/news/2025/04/how-do-bird-nests-stay-together
- https://www.pnas.org/doi/10.1073/pnas.2401868122
ランダムロッドパッキングにおけるエンタングルメント転移 Entanglement transition in random rod packings
Yeonsu Jung, Thomas Plumb-Reyes, Hao-Yu Greg Lin, and L. Mahadevan
Proceedings of the National Academy of Sciences Published:February 21, 2025
DOI:https://doi.org/10.1073/pnas.2401868122
Significance
Entanglement is normally thought of as a result of wrapping around by flexible or branched individual constituents as exemplified in tangled headphone chords or entangling vines. Contrary to this common intuition, stiff and straight rods can also entangle themselves by virtue of contact and steric repulsion between long, slender rods. Our quantification of the emergence of this entanglement phase in rod packings and its origin from mesoscale interactions allows us to understand the structural stability of entangled rods. Our findings highlight the role of outliers in physical entanglement in rod packings and may be relevant for applications ranging from animal architectures to the design and control of reconfigurable architectures or active fabrics.
Abstract
Random packings of stiff rods are self-supporting mechanical structures stabilized by long-range interactions induced by contacts. To understand the geometrical and topological complexity of the packings, we first deploy X-ray computerized tomography to unveil the structure of the packing. This allows us to directly visualize the spatial variations in density, orientational order, and the entanglement, a mesoscopic field that we define in terms of a local average crossing number, a measure of the topological complexity of the packing. We find that increasing the aspect ratio of the constituent rods in a packing leads to a proliferation of regions of strong entanglement that eventually percolate through the system and correlated with a sharp transition in the mechanical stability of the packing. To corroborate our experimental findings, we use numerical simulations of contacting elastic rods and characterize their stability to static and dynamic loadings. Our experiments and computations lead us to an entanglement phase diagram which we also populate using published experimental data from pneumatically tangled filaments, worm blobs, and bird nests along with additional numerical simulations using these datasets. Together, these show the regimes associated with mechanically stable entanglement as a function of the statistics of the packings and loading, with lessons for a range of systems from reconfigurable architectures and textiles to active morphable filamentous assemblies.



